Total methods of projection (cont.)
Logistic growth (cont.)
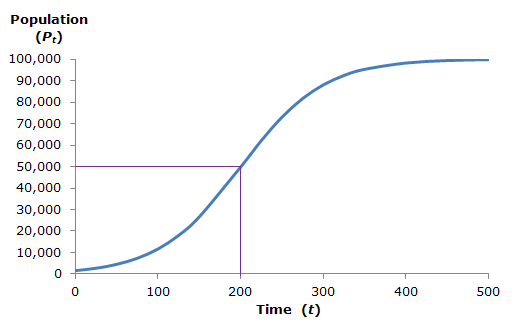
For example, in the diagram, at time 500 the population is close to achieving its final size of 100,000, having reached half that size at time 200.
Exercise
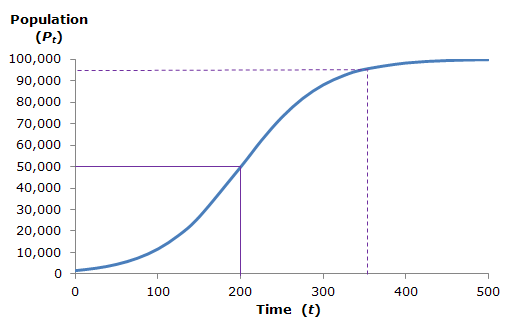
If s = 0.02, what is the projected size of the population at time 350?
Type your answer in the box below.
P(t) = P(∞) ⁄ (1 + e−s(t−h))
r = s(1 − P(t) ⁄ P(∞))
P(350) = 100,000 ⁄ (1+e−0.02(350−200)) = 100,000 ⁄ (1+e−3) = 95,257